Percent of increase word problems

Hello and welcome! We're focusing today on a fascinating mathematical topic: percent of increase word problems. We've got some exciting scenarios to consider and we'll walk through these calculations together. It'll be as simple as counting 1, 2, 3. Let's start!

Before we start, let's be clear on what percent of increase means. It's a way of expressing the growth of a quantity in terms of the original amount. Simply put, it’s a measure of how much something has grown as a proportion of the original size.

Problem #1

Let's consider a basic problem: If Jacob has 50 marbles and during a lucky streak, he wins 20 more. What is the percent of increase in his marbles?

First, figure out the increase - which is 20 marbles (the marbles he won). Then, divide this increase by the original quantity, which is 50 marbles. What do we get?

20 divided by 50 gives 0.4. To convert this into percentage, simply multiply this ratio by 100. Thus, you will get 40%. So, Jacob's marbles increased by 40%.

More percent of increase word problems

Problem #2

Great job so far! Let's try another. Imagine, you used to earn $30,000 yearly. After a fantastic performance review, your income increased to $36,000. What is the percent of increase?

Again, find the increase: $36,000 - $30,000 equals $6,000. Then divide this increase by the original amount, $30,000. Let’s see the magic!

$6,000 divided by $30,000 equals 0.2. Multiplying by 100 gives you a 20% increase in your earnings. Congratulations on your raise!

Problem #3

Feeling confident? Let's not stop there. What if you had 500 Instagram followers and over a month, you gained 75 more. What's the percent of increase in your followers?

Find the increase, which here is 75 followers. Divide this by the original quantity, 500 followers.

The good news? 75 divided by 500 is 0.15. After converting into a percentage, you will find there's been a 15% increase in your followers. Your popularity is increasing!

You're putting in some excellent work here! So, let's step things up a little.

Problem #4

Say, the population of a small town was 20,000 but over the decade it increased to 22,500. What's the percent increase in its inhabitants?

You've got this! The increase is 2,500 people. Divide this by the original population, 20,000 and let’s see what you get.

Amazing! 2,500 divided by 20,000 gives us 0.125. Converting this into a percentage results in a 12.5% increase in the town’s population. The town is growing!

Hopefully, these examples demystify the concept of percent of increase for you. Remember, understanding the underlying calculation rather than rote memorization will make this concept easy to understand and not confusing.

The percent increase is a very handy tool in mathematics. Whether it's analyzing your progress, calculating economic growth, personalized budgeting, or just winning at board games, knowing how to calculate a percent of increase will serve you in good stead.

These percent of increase problems clear up any mystery around this concept, and hopefully, have made it less daunting. They show that math involves logical thinking, step-by-step solutions, and best of all - it's completely solvable!

Practice makes perfect! So, feel free to create your own problems and use the steps we've reviewed to find your answers.

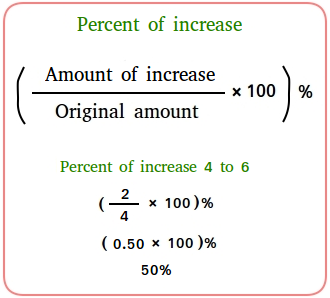
Always remember: the formula for percent of increase as shown above is (Amount of increase / Original Amount) × 100. Keep this in mind as you tackle new challenges.

In conclusion, no matter where you're starting or what you're working on, understanding percent of increase helps us track growth and progress. So next time you encounter a percent of increase word problem, take a deep breath, apply the steps, and solve it with confidence. You've got this!