Cavalieri's principle

The Cavalieri's principle states that if two or more figures have the same cross-sectional area at every level and the same height, then the figures have the same volume.

Take a close look at the figure below and make the following observations

• The area of each shaded cross section (shown in red) is 30.

• For the two prisms, the area is equal to 5 times 6 = 30

• For the two cylinders, the area is equal to 3.14 times 3.09 times 3.09 = 29.99 and 29.99 is very close to 30.

• Since the shapes all have the same height, their volume will be the same according to the Cavalieri's principle. 

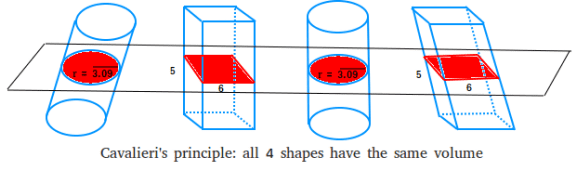
The geometric figures do not need to be the same type

You could move the plane in black higher or lower. As long as the area of the cross-sectional area is the same and the height is the same, the volume will be the same.

Cavalieri's principle and a stack of print paper.

The Cavalieri's principle makes perfect sense when you are looking at two stacks of print paper.

The stack on the left is a right prism and the stack on the right is an oblique prism. I made sure that both stacks have the same height by having the same amount of paper in both stacks.

The area of every cross section is the area of 1 sheet of paper. A sheet of paper right in the middle of the stack on the left will have the same area as a sheet of paper right in the middle of the stack on the right. Therefore the stacks must have the same volume.