Difference between linear speed and angular speed

Before you see the difference between linear speed and angular speed, you need to understand what linear speed is.

Let us spend some time talking about linear speed. The linear speed is simply the distance an object moves in a given time.

The linear speed is basically the same as the speed we defined in this lesson. The only difference between speed and linear speed is that the expression linear speed is used when the motion is circular.

Let us pretend that the circle below shows the view from the top of a merry-go-round.

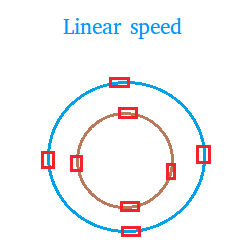
The red circles represent the horses. Notice that a horse moving around the blue circle have a greater linear speed than a horse moving around the brown circle. Can you tell why this is so?

Explanation:

The radius of the blue circle is bigger than the radius of the brown circle. As a result, the distance around the blue circle is bigger than the distance around the brown circle.

However,  the time it takes to complete a 360 degrees rotation or cycle is the same for both circles.

Suppose it takes the merry-go-round 30 seconds to complete a cycle. Suppose also that the distance around the blue circle is 60 feet and the distance around the brown circle is 40 feet.

Now, you are ready to understand what angular speed is. The owner of the merry-go-round is not going to let you ride forever. They may give you only 10 complete revolutions or cycles. The owner may also decide how fast the ride will complete these 10 cycles. 

An owner may set the ride to complete 10 cycles in 60 seconds or 1 cycle in 6 seconds. A different owner may set the ride to 1 cycle every 8 seconds, and so forth.

The number of cycles or revolutions in a given time is called angular speed.

What is the difference then between linear speed and angular speed?

All horses on a merry-go-round no matter where they are located have the same angular speed. However, those located on circles with bigger radii will have bigger linear speed.

Is there a formula?

We saw before that the linear speed depends on the radius of the circle. The bigger the radius, the bigger the linear speed. The linear speed also depends on the angular speed. A merry-go-round with angular speed of 20 RPM will have a greater linear speed than a merry-go-round with an angular speed of 5 RPM. 

Think of a cyclist. The faster the wheels of the bicycle revolve, the more distance the cyclist will cover which in turn will make the linear speed greater.

Suppose we have a rotating system. Let r represents the radius of a circle, let w the angular speed of the system, and let v represents the linear speed. Then, v is directly proportional to r and w. In other words both r and w will make v bigger.

The formula that shows this relationship is v = r × w.