Solving absolute value inequalities
When solving absolute value inequalities, the process is very similar to solving absolute value equations. You should review the latter before studying this lesson.
Solving absolute value inequalities using the definition of absolute value.
Absolute value definition:If x is positive, | x | = x
If x is negative, | x | = -x
Example #1:
Solve for x when | x | < 8
After applying the definition to example #1, you will have two equations to solve.
In fact, when solving absolute value inequalities, you will usually get two solutions. That is important to keep in mind!
If x is positive, | x | = x, so the first equation to solve is x < 8. This is done since x is automatically isolated.
If x is negative, | x | = -x, so the second equation to solve is -x < 8.
You can write -x < 8 as -1x < 8 and divide both sides by -1 to isolate x.
(-1/-1)x > 8/-1
1x > 8/-1
x > -8
Notice that the smaller sign (<) was switched to a bigger sign (>). This happens whenever you divide or multiply inequalities by a negative number.
We know that 2 < 4. However, let us divide both sides by a negative number, say -2
2/-2 ? 4/-2
-1 ? -2
Should ? be < or >?
Since -1 is bigger than -2, it should be -1 > -2
So, the solutions are x < 8 and x > -8
x > -8 means the same thing as -8 < x
Putting -8 < x and x < 8 together, you can write -8 < x < 8
Therefore, any number between -8 and 8 is a solution.
for instance, if we choose -5, we get | -5 | = 5 and 5 is smaller than 8.
Example #2:
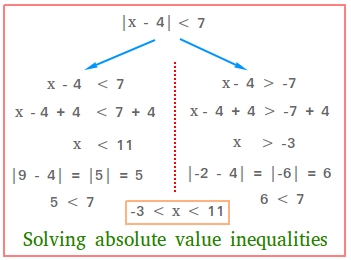
Solve for x when | x − 4 | < 7
Before, we apply the definition, let's make a useful substitution
Let y = x − 4, so | x − 4 | < 7 becomes | y | < 7. You must understand this step. No excuses!
Now, let's apply the definition to | y | < 7. Again, you will have two inequalities to solve
Once again, when solving absolute value inequalities, you will usually get two solutions.
If y is positive, | y | = y, so the first equation to solve is y < 7. No, you are not done! You have to substitute x − 4 for y
After substitution, y < 7 becomes x − 4 < 7
x − 4 < 7
x + -4 < 7
x + -4 + 4 < 7 + 4
x < 11
If y is negative, | y | = -y, so the second equation to solve is -y < 7.
You have to substitute x − 4 for y
You get -( x − 4) < 7. Notice the inclusion of parenthesis this time
-(x − 4) < 7
-(x + -4) < 7
Multiplying both sides by -1
-1 × -(x + -4) > -1 × 7 (Change < to >)
x + -4 > -7
x + -4 + 4 > -7 + 4
x > -3
The solutions are x > -3 and x < 11
This is equivalent to -3 < x < 11
Example #3:
Solve for x when | 3x + 3 | > 15
Before, we apply the definition, let's make a useful substitution.
Let y = 3x + 3, so | 3x + 3 | > 15 becomes | y | > 15.
Now, let's apply the definition to | y | > 15.
Lastly, when solving absolute value inequalities, you will usually get two solutions. We may never say this enough!
If y is positive, | y | = y, so the first equation to solve is y > 15. You have to substitute 3x + 3 for y
After substitution, y > 7 becomes 3x + 3 > 15
3x + 3 > 15
3x + 3 − 3 > 15 − 3
3x > 12
(3/3)x > 12/3
x > 4
If y is negative, | y | = -y, so the second equation to solve is -y > 15.
After substitution, -y > 7 becomes -(3x + 3) > 15
-(3x + 3) > 15
-3x + -3 > 15
-3x + -3 + 3 > 15 + 3
-3x > 18
(-3/-3)x > 18/-3
x < -6
The solutions are x > 4 and x < -6
Solving absolute value inequalities should be straightforward if you follow my guidelines above