Subtracting Fractions with the Same or Different Denominators
Subtracting fractions is easier than you think since it is very similar to adding fractions. Just like adding fractions, you need to keep in mind the following four important rules. Take the quiz to see if you really understand how to subtract fractions.
Fractions Quiz
Quiz completed 0 times
- You cannot subtract the denominators, also called bottom numbers!
- You can only subtract the numerators, also called top numbers!
- You can subtract the numerators only when the denominators are the same!
- If the denominators are not the same, look for a common denominator before subtracting the numerators.
How to Subtract Fractions with like Denominators
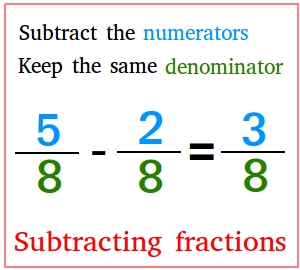
As shown in the figure above, the subtraction of 2/8 from 5/8 is simple. Since the denominator is the same, just subtract the numerators.
5/8 - 2/8 = (5 - 2)/8 = 3/8
A couple more examples showing how to subtract fractions with the same denominator
Example #1:
There is no need to look for a common denominator because they are already the same.
Example #2:
9/4 - 3/4 = (9 - 3)/4 = 6/4
How to subtract fractions with different denominators
Just like adding fractions, if the denominators are different, you will first find equivalent fractions that give a common denominator for both fractions.
Example #3:
Here the denominators are not the same, so multiply 3 by 2 to get the same denominator.
Remember that we have to multiply 7 by 2 also in order not to change the problem.
Example #4:
How to subtract fractions with whole numbers
Here are the three simple steps to follow when subtracting fractions with whole numbers.
Step 1
Convert the whole number into a fraction. You do this by using 1 as a denominator for the whole number.
Step 2
Look for the lowest common denominator. You just need to multiply 1 and the other denominator to get the lowest common denominator.
Step 3
Multiply the numerator and the denominator of the fraction in step 1 by the lowest common denominator.
Step 4
Subtract the fractions.
Example #5:
Subtract: 5 - 1/3
5 - 1/3 = 5/1 - 1/3 = 15/3 - 1/3 = (15 - 1)/3 = 14/3
Subtracting fractions and mixed numbers
When subtracting fractions with mixed numbers, just convert the mixed number into an improper fraction before subtracting.
Example #6:
7/3 - 1 2/3 = 7/3 - [(3 × 1 + 2) / 3]
7/3 - 1 2/3 = 7/3 - (5/3)
7/3 - 1 2/3 = (7 - 5)/3
7/3 - 1 2/3 = 2/3
If you don't know fractions very well, you will probably struggle to do well on most math tests. Build a strong foundation in math today before it is too late!
Buy my fractions ebook now. It offers a thorough coverage of fractions!
