Dividing Fractions
Dividing fractions is not hard. Instead, it is very straightforward once you know how to multiply fractions. It is important to explain some important terms or concepts before showing you how to divide fractions. Take the interactive quiz to see how much you know!
Fractions Quiz
Quiz completed 0 times
2/3 is a simple fraction and in the lesson about fractions, we called 2 the numerator and 3 the denominator. You can also call 2 the dividend and 3 the divisor. Dividend and divisor are mostly used when doing long division.
However, 3/4 ÷ 1/8 is a complex fraction.
When we divide complex fractions, the terms (dividend and divisor or numerator and denominator) can be used as well to describe the problem. However, quite often, we use the expressions "first fraction" for the fraction on the left (or on top) and "second fraction" for the fraction on the right (or at the bottom)
If you need to know more about complex fractions, check this lesson about complex fractions for a more comprehensive coverage. First, study this example.
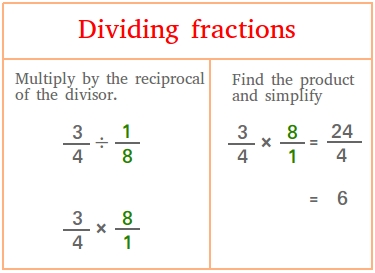
As shown in the figure above, here is how to divide fractions with four simple steps!
Step 1: Keep the first fraction the same.
Step 2: Change the division sign into a multiplication sign.
Step 3: Take the reciprocal of the second fraction.
Step 4: Multiply the numerators, multiply the denominators, and simplify.
Summary:
When you divide a fraction by another, invert the divisor (second fraction) and multiply the inverted divisor by the dividend (first fraction).
The inverted divisor is called the reciprocal. Inverting a fraction means that your numerator will become your denominator and your denominator will become your numerator. For example, when inverting 2/3, we get 3/2.
Note that instead of saying reciprocal of the second fraction it is quite common to say, flip the second fraction.
More Examples Showing how to Divide Fractions
Example #1:
3/4 ÷ 1/8
Here with this complex fraction, the dividend is 3/4 and the divisor is 1/8. Invert the divisor, change division into multiplication, and multiply.
3/4 ÷ 1/8 = 3/4 × 8/1
3/4 ÷ 1/8 = (3 × 8) / (4 × 1)
3/4 ÷ 1/8 = 24 / 4
3/4 ÷ 1/8 = 6
Did you notice that this is the same problem as the one in the figure above? Here though, we write the fraction with a '/' called 'slash' instead of a horizontal fraction bar.
Moreover, what you have is a fraction divided by another fraction.
Whenever a fraction is divided by another, we call this a complex fraction. This is a very simple example of complex fraction. Complex fractions can look a lot more complicated than this.
Example #2:
2/5 ÷ 4/3
2/5 ÷ 4/3 = 2/5 × 3/4
2/5 ÷ 4/3 = (2 × 3)/(5 × 4)
2/5 ÷ 4/3 = 6/20
To simplify 6/20, get the greatest common factor of 6 and 20.
GCF(6,20) = 2
Divide both numerator and denominator by 2.
You get 3/10
Example #3:
3/8 ÷ 3/4
3/8 ÷ 3/4 = 3/8 × 4/3
3/8 ÷ 3/4 = (3 × 4)/(8 × 3)
3/8 ÷ 3/4 = 12/24
3/8 ÷ 3/4 = 1/2
How to Divide Fractions by Whole Numbers
When dividing a fraction by a whole number or dividing a whole number by a fraction, the main thing to do is to convert the whole number into a fraction before following the steps outlined above.
Example #4:
4/10 ÷ 5
4/10 ÷ 5/1 = 4/10 × 1/5
4/10 ÷ 5/1 = (4 × 5)/(10 × 1)
4/10 ÷ 5/1 = 20/10
4/10 ÷ 5/1 = 2
What is the Common Denominator Method for Dividing Fractions?
The common denominator method is a shortcut or method that you can use to quickly divide fractions. If fraction #1 has the same denominator as fraction #2, then you can just divide the numerator of fraction #1 by the numerator of fraction #2 to get an answer.
Example #5:
4/8 ÷ 2/8 = 4/2 = 2
As you can see, you can get the answer very fast as opposed to following the steps.
4/8 ÷ 2/8 = 4/8 × 8/2
4/8 ÷ 2/8 = (4 × 8)/(8 × 2)
4/8 ÷ 2/8 = 32/16
4/8 ÷ 2/8 = 2