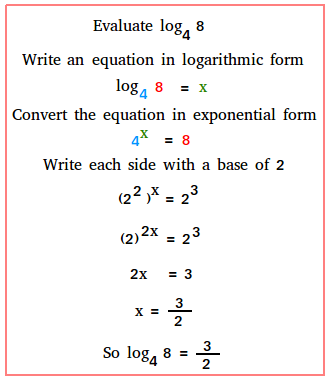
How to evaluate logarithms
Recommended Lessons
Not sure where to start? These are the lessons and games we recommend most to students, parents and teachers on Basic-mathematics.com. Each lesson explains the topic in plain language, walks you through worked examples step by step, and ends with practice so you can check your understanding. Pick one below, or use the menu to find lessons on fractions, algebra, geometry, statistics and more.
This lesson will show how to evaluate logarithms with some good examples. Study the first example below carefully.
Examples showing how to evaluate logarithms
Example #1:
Evaluate log8 16
Write an equation in logarithmic form
log8 16 = x
Convert the equation to exponential form
16 = 8x
Write each side using base 2.
24 = (23)x
24 = 23x
Since the base or 2 is the same, 24 = 23x if 4 is equal to 3x
If 4 = 3x, then x = 4/3
Therefore, log8 16 = x = 4/3
Example #2:
Evaluate log10 1000
Write an equation in logarithmic form
log10 1000 = x
Convert the equation to exponential form
1000 = 10x
Write each side using base 10.
103 = (10)x
Since the base or 10 is the same, 103 = 10x if 3 is equal to x
Therefore, log10 1000 = x = 3
Example #3:
Evaluate log64 1/16
Write an equation in logarithmic form
log64 1/16 = x
Convert the equation to exponential form
1/16 = 64x
Write each side using base 4.
1/42 = (43)x
4-2 = 43x
Since the base or 4 is the same, 4-2 = 43x if -2 is equal to 3x
If -2 = 3x, then x = -2/3
Therefore, log64 1/16 = x = -2/3
Example #4:
Evaluate log5 (-25)
Write an equation in logarithmic form
log5 (-25) = x
Convert the equation to exponential form
-25 = 5x
No matter what x is, you could never get 5x to equal to -25!
The logarithm of a negative number does not exist as a real number!
How to evaluate logarithms when it is not possible to write each side of the equation with the same base.
Example #5:
Evaluate log3 10
Write an equation in logarithmic form
log3 10 = x
Convert the equation to exponential form
3x = 10
Now, as you can see it is not possible to write each side with a base of 3 since it is very hard to rewrite 10 with a base of 3.
What we can do is to take the common logarithm of each side
log10 3x = log10 10
What is log10 10 equal to?
In logarithmic form, log10 10 is log10 10 = y
In exponential form, log10 10 = y is 10y = 10 or 10y = 101
So y = 1 and log10 10 = 1
Substitute 1 for log10 10 in log10 3x = log10 10
We get log10 3x = 1
x log10 3 = 1
x = 1/(log10 3)
x = 1/0.477
x = 2.096
log3 10 = 2.096
Now study carefully the following figure with summarizes the concept
