Substitution method
Recommended Lessons
Not sure where to start? These are the lessons and games we recommend most to students, parents and teachers on Basic-mathematics.com. Each lesson explains the topic in plain language, walks you through worked examples step by step, and ends with practice so you can check your understanding. Pick one below, or use the menu to find lessons on fractions, algebra, geometry, statistics and more.
System of linear equations, also called simultaneous equations, can also be solved using the substitution method. This lesson will show how to solve a pair of linear equations with two unknown variables.
ax + by = c
dx + ey = f
Before you read this lesson, make sure you understand how to solve linear equations.
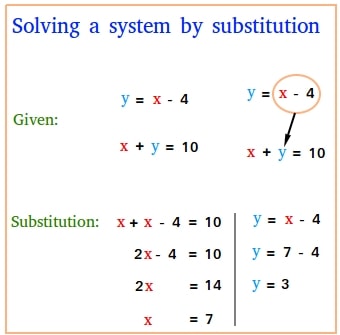
Steps to follow to solve a system of linear equations by substitution
- Pick an equation and solve either for x or for y. It may be beneficial to pick the equation where it is easy to solve for the variable.
- If you solved for x in step 1, substitute the value of x into the other equation. Otherwise, if you solved for y, substitute the value of y into the other equation.
- If you solved for x in step 1 and substituted the value of x into the other equation, you will end up with a linear equation where the variable is y. Solve for y!
If on the other hand, you solved for y in step 1 and substituted the value of y into the other equation, you will end up with a linear equation where the variable is x. Solve for x! - Plug the value of the variable you found in step 3 into one of the original equations in order to find the value of the other variable.
Examples showing how to solve a system of equations using the substitution method
Example #1: Solve the following system using the substitution method
x + y = 20
x − y = 10
Step 1
You have two equations. Pick either the top equation or the bottom equation and solve for either x or y.
Since I am the one solving it, I have decided to choose the equation at the bottom (x − y = 10) and I will solve for x.
x − y = 10
Add y to both sides
x − y + y = 10 + y
x = 10 + y
Step 2
Since you used the equation at the bottom and solved the equation for the variable x, you will substitute x into the equation on top (x + y = 20)
Using x + y = 20, erase x and write 10 + y since x = 10 + y
We get 10 + y + y = 20
Step 3
Solve for y
10 + y + y = 20
10 + 2y = 20
Minus 10 from both sides
10 − 10 + 2y = 20 − 10
2y = 10
Divide both sides by 2
y = 5
Step 4
Since you have found the value of the variable y, you can plug its value into either the top equation or the bottom to get x.
Replacing y into x + y = 20 gives x + 5 = 20
Minus 5 from both sides
x + 5 − 5 = 20 − 5
x = 15
The solution to the system is x = 15 and y = 5
Indeed 15 + 5 = 20 and 15 − 5 = 10
Example #2: Solve the following system using the substitution method
3x + y = 10
-4x − 2y = 2
Step 1
You have two equations. Pick either the first equation (top) or the second equation (bottom) and solve for either x or y.
I have decided to choose the equation on top (3x + y = 10) and I will solve for y.
3x + y = 10
Subtract 3x from both sides
3x − 3x + y = 10 − 3x
y = 10 − 3x
Step 2
Since you used the equation on top to solve for y, you will substitute y into the equation at the bottom (-4x − 2y = 2)
Using -4x − 2y = 2, erase y and write 10 − 3x keeping in mind that there is a multiplication between 2 and y.
We get -4x − 2×(10 − 3x ) = 2
Step 3
Solve for x
-4x − 2 ×(10 − 3x ) = 2
-4x − 20 + 6x = 2 (After multiplying -2 by 10 and -2 by -3x)
2x − 20 = 2
Add 20 to both sides
2x − 20 + 20 = 2 + 20
2x = 22
Divide both sides by 2
x = 11
Step 4
Now you have x, you can replace its value into either the first equation on top or the second equation at the bottom to get y.
Replacing x into 3x + y = 10 gives 3 × 11 + y = 10
33 + y = 10
Minus 33 from both sides
33 − 33 + y = 10 − 33
y = -23
The solution to the system is x = 11 and y = -23
Indeed,
3 × 11 + -23 = 33 + -23 = 10 and -4 × 11 − 2 × -23 = -44 + 46 = 2
You should have noticed so far that the reason we call this method the substitution method is because after you have solve for a variable in one equation,you substitute the value of that variable into the other equation.
Using the substitution method to show that a system of equations has infinitely many solutions or no solution
Example #3: Solve the following system using the substitution method
2x + y = 8
2x + y = 8
Step 1
Pick the equation on top and solve for y.
2x + y = 8
2x - 2x + y = 8 - 2x
y = 8 - 2x
Step 2
Substitute the value of y in the equation at the bottom.
2x + 8 - 2x = 8
8 = 8
Notice that instead of finding a value for x, we end up with a true statement (8 = 8)
When this happens, it means that the system has an infinite number of solutions. Any ordered pair (x,y) that solves the system is a solution. Find ordered pairs by choosing anything you want for x and then solve for y.
For example, if I choose 1 for x, then 2(1) + y = 8
2 + y = 8
y = 6
A solution is (1, 6). You can find an infinite amount if you keep choosing arbitrary value for x.
If you solve a system by substitution and you end up with a true statement, it means that the system has an infinite number of solutions.
Example #4: Solve the following system using the substitution method
2x + y = 4
2x + y = 8
Step 1
Pick the equation on top and solve for y.
2x + y = 4
2x - 2x + y = 4 - 2x
y = 4 - 2x
Step 2
Substitute the value of y in the equation at the bottom.
2x + 4 - 2x = 8
4 = 8
Notice that instead of finding a value for x, we end up with a false statement (4 = 8)
If you solve a system by substitution and you end up with a false statement, it means that the system has no solution.