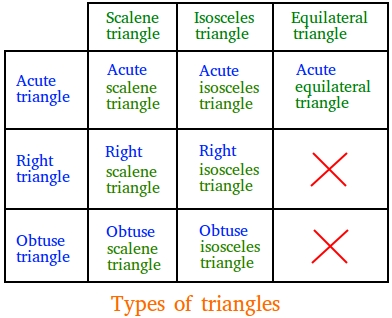
Types of Triangles

Recommended Lessons

Not sure where to start? These are the lessons and games we recommend most to students, parents and teachers on Basic-mathematics.com. Each lesson explains the topic in plain language, walks you through worked examples step by step, and ends with practice so you can check your understanding. Pick one below, or use the menu to find lessons on fractions, algebra, geometry, statistics and more.

Types of Triangles

The different types of triangles may be classified by their side lengths, by their internal angles or by a combination of both their side lengths and their internal angles. Basically, there are six types of triangles that can be classified either by their sides or by their angles.

In this lesson, you will learn

  • the three types of triangles by sides: scalene, isosceles and equilateral
  • the three types of triangles by angles: acute, right and obtuse
  • how to name a triangle using its sides and its angles together
  • which combinations are impossible, such as a right equilateral triangle

Types of Triangles Classified by Their Sides Only

A triangle classified by its sides only can either be scalene, isosceles, or equilateral.

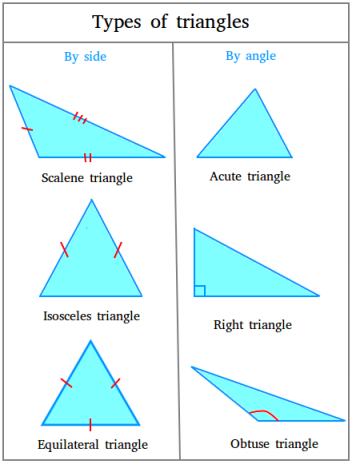
Scalene triangle

A scalene triangle is a triangle that has no equal sides.

Scalene triangle with 1, 2 and 3 red marks on its sides, showing that all three sides have different lengths.

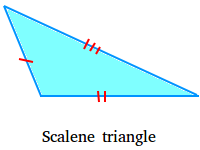
Notice the use of red marks (1, 2 and 3 marks) to show that the lengths of the sides are not the same.

Isosceles triangle

An isosceles triangle is a triangle that has two equal sides.

Isosceles triangle with 1 red mark on each of its two equal sides.

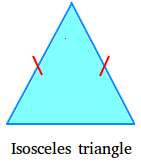
Notice the use of 1 red mark on each of the two sides that are equal.

Equilateral triangle

An equilateral triangle is a triangle that has three equal sides.

Equilateral triangle with 1 red mark on each of its three equal sides.

Notice the use of 1 red mark on each of the three sides that are equal.

A triangle has sides of 7 cm, 7 cm and 4 cm. What type of triangle is it by its sides?

Show answer

Isosceles. Two of its sides are equal (7 cm and 7 cm).

Types of Triangles Classified by Their Angles Only

A triangle classified by its angles only can either be acute, right, or obtuse.

Right triangle

A right triangle, also called right-angled triangle, has a 90-degree angle.

Right triangle with a small square in one corner showing the right angle.

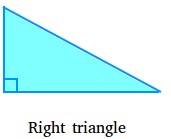
Notice that we use a small square to indicate that there is a right angle there.

Obtuse triangle

An obtuse triangle has only one angle that is bigger than 90 degrees (an obtuse angle).

Obtuse triangle with its obtuse angle marked in red.

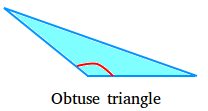
Notice that the angle that is obtuse is shown in red. The other two angles are acute angles.

Acute triangle

In an acute triangle, also called acute-angled triangle, all angles are less than 90 degrees, so all angles are acute angles.

Acute triangle: all three angles are less than 90 degrees.
Remember

The three angles of a triangle add up to 180°. So a triangle can have only one right angle or one obtuse angle; the other two angles are always acute.

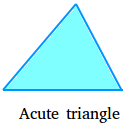
Figure Summarizing the Six Different Types of Triangles Based Either on Their Sides or Their Angles

Types of triangles. By side: scalene, isosceles and equilateral triangles. By angle: acute, right and obtuse triangles.

The angles of a triangle are 50°, 60° and 70°. What type of triangle is it by its angles?

Show answer

Acute. All three angles are less than 90°.

Classifying Triangles Based on Their Sides and Their Angles

We can also name triangles using angles and sides at the same time. You will end up with 7 more types.

  • If a triangle has only acute angles and no equal sides, we can call that triangle acute scalene triangle.
  • If a triangle has only acute angles and two equal sides, we can call that triangle acute isosceles triangle.
  • If a triangle has three equal sides and only acute angles, we can call that triangle acute equilateral triangle.
  • If a triangle has one right angle and no equal sides, we can call that triangle right scalene triangle.
  • If a triangle has one right angle and two equal sides, we can call that triangle right isosceles triangle.
  • If a triangle has two equal sides and one obtuse angle, we can call that triangle obtuse isosceles triangle.
  • If a triangle has no equal sides and one obtuse angle, we can call that triangle obtuse scalene triangle.

Study also the figure below summarizing the seven types of triangles based on their sides and angles.

Table of the seven types of triangles. Rows acute, right and obtuse; columns scalene, isosceles and equilateral. Right equilateral and obtuse equilateral are crossed out because they are impossible.
Common mistake

Thinking a right triangle cannot also be isosceles. It can: a right triangle with two equal sides is a right isosceles triangle, and its angles are 90°, 45° and 45°.

A triangle has an angle of 120° and no equal sides. Name it using its sides and angles.

Show answer

Obtuse scalene triangle. 120° is an obtuse angle, and no sides are equal.

Examples on Types of Triangles

Example #1: The lengths of the sides of a triangle are 8 cm, 5 cm, and 6 cm. Name the triangle.

Since all the sides are of different lengths, the triangle is a scalene triangle.

Example #2: The lengths of the sides of a right triangle are 12 inches, 12 inches, and about 17 inches. Name the triangle.

The triangle is a right triangle and two sides are the same. Therefore, the triangle is a right isosceles triangle.

Example #3: The lengths of the sides of a triangle are 7 cm, 3 cm, and 5 cm and the triangle has an angle that is equal to 120 degrees. Name the triangle.

The triangle has an obtuse angle and the three sides are not the same. Therefore, the triangle is an obtuse scalene triangle.

Worked example: A triangle has two equal sides and angles of 40°, 40° and 100°. Name it using its sides and angles.
  1. Sides: two sides are equal, so it is isosceles.
  2. Angles: 100° is bigger than 90°, so it is obtuse.
  3. Put the two names together, angle name first.

Answer: obtuse isosceles triangle

Is it possible to have an obtuse equilateral triangle?

No, it is not possible! A triangle cannot be obtuse and equilateral at the same time. An equilateral triangle cannot have an obtuse angle because all 3 angles in an equilateral triangle measure 60 degrees.

Is it possible to have a right equilateral triangle?

No. If the triangle is a right triangle, then one of the three angles is equal to 90 degrees. The sum of the other two angles must then add up to 90 degrees. This makes it impossible for all three angles to be equal.

Can a triangle have two right angles?

Show answer

No. 90° + 90° = 180°, which would leave 0° for the third angle.

Summary

  • By sides: scalene (no equal sides), isosceles (two equal sides), equilateral (three equal sides).
  • By angles: acute (all angles less than 90°), right (one 90° angle), obtuse (one angle bigger than 90°).
  • Equal sides are shown with matching red marks, and a right angle with a small square.
  • You can name a triangle by both, angle name first: for example, right isosceles triangle.
  • Right equilateral and obtuse equilateral triangles are impossible, because each angle of an equilateral triangle is 60°.

Test Yourself: 10-Question Quiz

Now check what you learned. Every answer is explained, and each time you play you get new questions and new triangles.

Question 1 of 10Score: 0

Keep learning